|
|||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||
|
Feature Articles: Toward Quantum Technology Innovation Vol. 21, No. 6, pp. 53–57, June 2023. https://doi.org/10.53829/ntr202306fa7 Toward a Quantum InternetAbstractA quantum internet holds promise for accomplishing quantum metrology and quantum computer networks as well as quantum communication among arbitrary clients all over the globe. Its actualization is an important long-term scientific and technological goal. In this article, I explain what a quantum internet is and what is needed for its actualization as well as recent relevant progress in the field of quantum information. Keywords: quantum internet, quantum repeaters, all-photonic approach 1. IntroductionIn modern physics, quantum mechanics gives the most precise description of nature in the range of phenomena from the level of elementary particles to our universe. Quantum mechanics just predicts the probability of an event occurring, in contrast to the determinism given by classical mechanics, and it is a broader paradigm than classical mechanical views. Even if there is a theory with the view of determinism, quantizing it is still one of most important attempts in physics. At the end of the last century, the possibility of applying the quantum mechanical point of view to information processing began to be explored; as a result, quantum information processing was established. Quantum information processing accomplishes tasks that are intractable by conventional means and the concept includes the paradigm of conventional information processing [1]. For instance, a quantum computer can factor large integers efficiently. Thus, if a quantum computer is handed to an eavesdropper, most widely used public-key cryptosystems, such as RSA (Rivest–Shamir–Adleman), can be cracked. Quantum key distribution (QKD), however, presents information-theoretically secure communication even if eavesdroppers can use arbitrary attacks enabled by quantum mechanics (including eavesdropping by using an arbitrarily large quantum computer). As long as quantum mechanics is correct in the description of nature, such quantum information processing allowed by the laws of quantum mechanics must be the ultimate form of achievable information processing. What is the ultimate form of quantum information processing? Given that the current internet could be regarded as the largest information-processing network on Earth, its quantum version, called a quantum internet [2], would be the ultimate form of information-processing networks. In this article, I explain what a quantum internet is and what is needed for its actualization as well as recent relevant progress in the field of quantum information (see review paper [3] for detail). 2. What is a quantum internet?A quantum internet is a quantum-information-processing network (Fig. 1) in which quantum-information-processing nodes (such as quantum computers and quantum memories) are connected by quantum communication channels (such as optical fibers and free space). It enables arbitrary clients around the globe to achieve various quantum-information-processing tasks beyond those served by the current internet [4]. For example, it enables arbitrary clients in the network to use QKD, which could be the basis of a referendum, top-level meeting, financial deal, exchange of genetic/biological information and so on, thanks to its feature of high security. A quantum internet would also make it possible to transfer unknown quantum states faithfully to a distant place at the speed of light. This is the basis of distributed quantum computation, cloud quantum computing, and networking of quantum computers. A quantum internet would also be used for synchronizing atomic clocks with unprecedented stability and accuracy in a secure manner. It would also enable us to make baselines of telescope arrays unprecedentedly longer, contributing to progress of astronomy.
3. Constructing a quantum internetHow can we construct a quantum internet? The role of a quantum internet is to distribute quantum entanglement to clients efficiently. Quantum entanglement can be demonstrated only with quantum systems such as atoms and photons, and it is a peculiar correlation that cannot be explained in the framework of classical mechanics. This correlation was used by Einstein, Podolsky, and Rosen to point out that quantum mechanics includes a prediction contradicting the local realism (which ought to hold in classical mechanics), that is, to pose a question on the validity of quantum mechanics [5]. Ironically, the existence of quantum entanglement has been confirmed experimentally. In 2002, Aspect, Clauser, and Zeilinger, who had conducted these experiments, were awarded the Nobel Prize in Physics [6]. Quantum entanglement is now identified as a universal resource not only for quantum communication but also for quantum computation. Therefore, by distributing entanglement as such a universal resource efficiently, a quantum internet will serve clients with various functions. Quantum networks for QKD have been developed worldwide, as exemplified by the SECOQC network in Europe [7], the Tokyo QKD network in Japan [8], and the 2000-km Shanghai-Beijing network in China [9]. However, all the nodes in these networks, including repeater nodes, are not quantum-information-processing nodes but classical information-processing nodes that can process only classical signals at best even if they receive quantum signals. Therefore, we cannot distribute quantum entanglement to arbitrary clients in those networks. Even if we specialize the use of the networks only for QKD, we cannot distribute a secret key unless we can fully trust all the nodes in the networks. In this regard, such existing networks are categorized as trusted-node networks, different from a quantum internet, and they cannot be used to actualize a quantum internet. If all clients are connected completely with quantum communication channels, that is, if all the clients can communicate with each other by using point-to-point quantum communication schemes, the network can work as a secure QKD network, which could be used to actualize a quantum internet. However, it is not realistic in terms of cost and efficiency if we actualize a quantum internet by expanding such a point-to-point quantum communication network worldwide. For example, if we use a standard optical fiber as the quantum communication channel, its transmittance decreases exponentially with its length. In particular, the probability with which a single-photon level of light emitted by a sender to convey quantum information is detected by a receiver is about 10% for a distance of 50 km, about 1% for 100 km, and about 0.1% for 150 km, decreasing as it is multiplied by 0.1 every 50 km. Therefore, if a sender and receiver execute point-to-point quantum communication with a 1000-km optical fiber, even with the use of a GHz clock system, the expectation time needed to establish an entanglement pair between them is on the order of hundreds of years, which is not realistic. Hence, it is impossible to actualize a quantum internet only by combining point-to-point quantum communication schemes. To do so, we need to construct a network in which quantum-information-processing nodes, called quantum repeaters, are installed between clients [10, 11]. 4. What is a quantum repeater?Even in conventional communication, if a sender and receiver are far apart, their communication is established not with point-to-point means but via repeater nodes set between them. In conventional communication, the role of classical repeaters located on the repeater nodes is to amplify the received weakened signal then send the amplified signal to the next repeater node (or the receiver if the repeater is neighboring the receiver). However, this method does not work for quantum communication because the quantum no-cloning principle forbids copying and amplifying quantum signals [12]. Thus, quantum repeaters do not rely on signal amplification, although they are located on the repeater nodes between a sender and receiver similarly to classical repeaters. The quantum repeater protocol based on the use of quantum repeaters is composed of two processes; entanglement generation and entanglement swapping. In a memory-based approach [10] (Fig. 2), quantum repeaters, equipped with matter quantum memories and quantum interfaces, are connected to each other by optical fibers. By exchanging photons between adjacent repeater nodes, we attempt to generate quantum entanglement between them and store the entanglement in quantum memories in the quantum repeaters once the attempt succeeds. By repeating this entanglement-generation process, if all the adjacent repeater nodes are connected with entanglement, we then move on to entanglement swapping. In this process, by applying a measurement—called the Bell measurement—to halves of entangled pairs, the entanglement pairs are transformed into an entangled pair that directly connects the sender and receiver. Therefore, in the memory-based approach, we can present entanglement between the sender and receiver by conducting entanglement generation, followed by entanglement swapping.
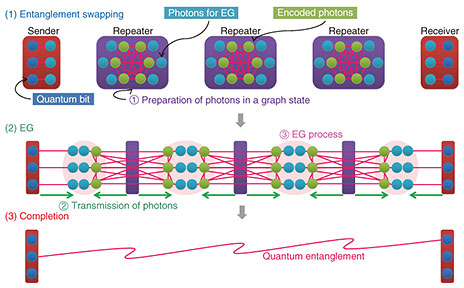
An all-photonic approach [11] is based on a time-reversed version of the memory-based repeater protocol, where we first carry out operations associated with entanglement swapping, followed by entanglement generation (Fig. 3). In this approach, all the repeater nodes first prepare photons in an entangled state, called a graph state, to execute entanglement swapping. The halves of the graph states are sent to neighboring repeater nodes. On receiving the halves, the receiving nodes apply entanglement generation to the received pulses and, depending on this result, execute measurements on remaining photons. With a high probability, an entangled pair is then presented to the sender and receiver. Quantum repeaters in this all-photonic approach do not necessitate matter quantum memories and quantum interfaces and the protocol works only with optical devices (such as single-photon sources, linear optical elements, active feedforward controllers, and photon detectors), in contrast to the memory-based approach. Therefore, its repetition rate does not depend on the communication distance and is determined only by the clock speed of optical devices. Therefore, the all-photonic approach has high affinity with the current all-optical trend in conventional communication, and the served communication speed will be faster.
5. DiscussionThanks to recent theoretical progress in the understanding of quantum networks (see review article [13]), the quantum capacity—the number of transmittable quantum bits per optical mode—and the private capacity—the number of securely transmittable bits per optical mode—of an optical fiber with a transmittance η are identified as −log2(1 − η). This describes the fundamental limit of the point-to-point quantum communication based on the use of an optical fiber, showing the impossibility of long-distance quantum communication as well as a quantum internet, without quantum repeaters (on considering η = e−l/latt for the length l of the fiber and a constant latt). The quantum/private capacity for two-party communication over any optical-fiber network with arbitrary topology was derived and achieved by aggregating quantum repeater protocols. This indicates that quantum repeaters play essential roles in establishing a quantum internet that achieves or approaches such ultimate performance. There is a technological and conceptual gap between currently available point-to-point QKD technology and quantum repeater technology necessary for a quantum internet. Exploring and implementing schemes to bridge this gap is now being investigated in QKD [14]. For instance, the adaptive measurement-device-independent QKD protocol and twin-field QKD protocol have been found to be candidates for bridging such a gap. Proof-of-principle experiments on quantum repeaters, with the all-photonic approach as well as the memory-based approach, have been conducted. For the memory-based approach, experiments of the working principle of entanglement swapping have been conducted with the use of quantum memories, such as silicon-vacancy color centers integrated inside a diamond nanophotonic cavity at Harvard University and Massachusetts Institute of Technology in the US [15], ensembles of Rubidium atoms at Tsinghua University in China [16], and nitrogen-vacancy centers in diamond at Delft University of Technology in the Netherlands [17]. For the all-photonic approach, experimental demonstration of the principle of time-reversed entanglement swapping has been carried out using an entangled graph state, called a Greenberger-Horne-Zeilinger state, generated through the parametric-down conversion process, at Osaka University, NTT, the University of Toyama, and the University of Toronto [18], and at the University of Science and Technology of China [19, 20].
|
|||||||||||||||||||||||||||||||||||||||||