Observation of Exceptional Point Degeneracy with Current-injected Photonic Crystal Lasers

1.1 PT symmetry

A turning point in these circumstances was the introduction of the concept of parity-time (PT) symmetry to photonics [1] by the group headed by Professor Christodoulides at the University of Central Florida. Parity in PT symmetry means spatial inversion. This corresponds to setting a central axis or plane of symmetry (double-sided mirror) in space then inverting the system (or jumping to the world on the other side of the mirror). Time in PT symmetry stands for time reversal, which means turning gain to loss and loss to gain. A system is said to have PT symmetry when both of these operations make it get back to its original shape. While PT symmetry has attracted attention in quantum mechanics, Makris et al. showed that the analogy of PT symmetry in the above-mentioned sense held in the refractive index distributions of optical systems. They also showed that such a system could have a peculiar mode degeneracy called an exceptional point (EP).

We now explain the characteristics of a coupled two-cavity system with PT symmetry shown in Fig. 1(a). We assume that each resonator has an eigenmode (cavity mode) with an identical resonance frequency when isolated. The cavities are placed in proximity with an interval of the order of the mode wavelength. Thus, a small number of fields of one cavity mode seeps into the other via the evanescent wave^*1 so that they are coupled with each other. The system is PT-symmetric when two cavities have loss and gain with the same magnitude γ. Note that the gain rate is denoted as a negative value of –γ, which is contrasted to that of the loss γ > 0.

1.2 EP phase transition

Figure 1(b) shows how the system response changes when the coupling rate is fixed to κ and the γ of the balanced gain and loss is varied. The left and right plots respectively show the eigenmode frequencies (the real part of the complex eigenfrequency) with reference to the single cavity mode frequency ω₀ and their net loss rates (the imaginary part of the complex eigenfrequency) for which negative values mean gain. When γ = 0, which corresponds to the Hermitian system, the two eigenmode frequencies are split by 2κ by the coupling. The eigenmode distributions are two orthogonal states termed symmetric and antisymmetric. As γ increases, the frequency splitting decreases then changes abruptly toward zero near γ = κ. In this case, because both eigenmodes distribute an equal field intensity for the two cavities with balanced gain and loss, their net loss or gain rates are exactly zero. When γ > κ, the two mode frequencies are the same. In contrast, the eigenmodes’ loss or gain rates split sharply into positive and negative values. Despite the fact that the cavities are supposed to be coupled, the eigenmodes become localized by the increase in γ; one concentrates in the cavity with gain, hence amplified, and the other is in the lossy cavity, thus damped. When the gain, loss, and coupling rates are equal (γ = κ), the eigenmode frequencies coincide, and the net loss or gain rates remain zero just before the bifurcation. What happens is not the accidental degeneracy of two different states with the same complex frequency; the distributions of the two modes rather become completely the same. Thus, there is only a single state. The degeneracy in this sense is a phenomenon peculiar to non-Hermitian systems and termed an EP. It can also be said that an EP is a phase-transition point from modes spreading over the entire system to those localized in cavities with gain or loss.

Fig. 1. PT symmetry and EP phase transition of two coupled resonators.

To date, a number of unconventional phenomena based on this EP phase transition have been reported, and readers can refer to a previous review article [2]. Examples include non-reciprocal propagation^*2, single-mode oscillation, and the enhancement of frequency perturbation effect, each of which has a good potential for practical use. Non-reciprocal propagation is a necessary condition for an optical isolator that protects a laser against instability by undesired optical feedback. Although integrating an optical isolator into a photonic circuit is technically difficult, it is an important element to complete optical information processing within a chip. Unfortunately, the non-reciprocal property near an EP is valid only for a certain range of the input light intensity because it is based on gain saturation. Thus, further studies are needed to pave the way for achieving a practical isolator based on an EP.

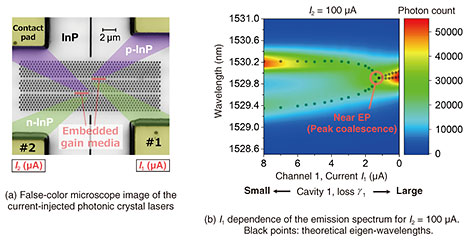
2.1 Current-injected photonic crystal laser

We fabricated the coupled system of two current-injected photonic crystal lasers [3] shown in Fig. 2(a). The system is structured on an indium phosphide (InP) thin film suspended in air. It has a two-dimensional photonic crystal with an array of air holes with a diameter of about 200 nm. Two wavelength-scale gain media^*3 with quantum wells (red rectangles in the figure) are embedded in line defects without air holes and work as nanocavities. In addition, p-doped layers (purple parts) and n-doped layers (green parts) are formed in the oblique directions from the four electrodes (yellow parts), constituting two in-plane current-injection channels. Because this structure suppresses the leakage current between the electric channels, the gain and loss of each nanocavity can be controlled independently by current injection. To date, by using such a photonic crystal laser on the basis of a buried heterostructure nanocavity, we demonstrated the smallest laser threshold current in the world [4]. The low current drive of our system minimalizes the detrimental effect of the cavity-frequency detuning due to heat and carriers.

In Fig. 2(a), the right and left cavities and current channels are numbered 1 and 2, and the inter-electrode current values for channel 1 and 2 are denoted as I₁ and I₂, respectively. The emission spectrum of the system was measured while I₁ and I₂ were varied. As a result, when I₂ was fixed at 100 μA and I₁ was decreased, a clear EP phase transition was observed. Figure 2(b) shows the color plot of such emission spectra for different I₁. Here, I₂ = 100 μA is the condition to compensate for the loss γ₂ due to absorption and radiation in cavity 2 (γ₂ ~ 0). However, a finite loss γ₁ of resonator 1 remains. Consequently, the entire system does not oscillate, and weak spontaneous emission of photons is hence observed. As I₁ diminishes, the two spectral peaks indicating coupled modes approach each other and eventually coalesce. This is because the difference in the loss of the two cavities is enhanced by the increase in γ₁, resulting in the variation of the eigenmode frequencies similar to that in Fig. 1(b). By fitting the spectra using a theoretical model, we can estimate the resonance frequency of each cavity mode, cavity coupling κ, and loss γ₁. We found that the system was very near the exact EP when I₁ = 1.4 μA. The detrimental frequency detuning of the two cavities is also very small. This can be seen from the fact that the theoretical eigen-wavelengths (black points in Fig. 2(b)) calculated from the fitting result are aligned with a point in the relevant region. If I₁ is further decreased, the peak photon number increases in spite of the magnified loss. This is thought to be because one of the eigenmodes is localized in resonator 2, which has a large injection current, becoming less susceptible to the loss of resonator 1. This intensity recovery also supports the EP phase transition illustrated in Fig. 1(b).

Fig. 2. EP phase transition of current-injected photonic crystal lasers.

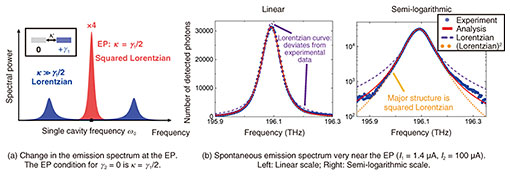
2.2 Spontaneous emission spectrum at the EP

We also clarified that the spectrum near the observed EP mentioned above indicated the peculiar enhancement of spontaneous emission. Even though the loss of one cavity is compensated and the other has a loss, the system with a large coupling exhibits a spontaneous emission spectrum that is expressed as the sum of two split Lorentzian functions^*4 (blue spectrum in Fig. 3(a)). In contrast, the emission spectrum of the EP state takes the shape of the squared Lorentzian function (red spectrum in Fig. 3(a)) [5]. When the system can be approximated as a Hermitian one, the two modes are almost independent. Thus, even if their peaks coincide accidentally, the emission spectrum will only be the sum of them, that is, a Lorentzian function with twice their peak intensity. In stark contrast, the two modes degenerate into exactly the same state at the EP, exhibiting constructive interference in the spectral domain. Suppose we vary only the coupling κ that always preserves the energy so that the system is dragged to the EP condition while keeping the other parameters constant. In this case, we can measure the impact of the spectral change induced purely by EP degeneracy. Because of the maximum intensity change due to the interference, the peak intensity of the EP spectrum (colored red) is four times (= (1 + 1)²) larger than those of the split Lorentzian peaks (blue). From the energy conservation, the total integrated powers for the two spectra in Fig. 3(a) are equal, and the loss of the eigenmodes for them is also the same. Despite this, the peak intensity of the EP is enhanced via the spectral change in which the shape becomes squared-Lorentzian; thus, the linewidth becomes narrower than the Lorentzian peaks for the large coupling.

Our system can control the gain and loss of each laser but not the coupling between the lasers. In our experiment, unfortunately, the effect of reducing the peak intensity by increasing γ₁ was superimposed on the measurement results. Thus, the directly observed ratio of the peak-intensity enhancement by the EP is about 30%. However, as shown in Fig. 3(b), we also observed that the emission spectrum at I₁ = 1.4 μA, which was closest to the EP, was a squared Lorentzian function. This result indicates that the system is under an almost exact EP degeneracy. This means that the effect of EP degeneracy can be clearly observed if the system approaches the states at which its complex-eigenfrequency splitting is small enough compared with the linewidths of the modes.

Fig. 3. Enhancement of the system’s spontaneous emission due to EP degeneracy.